So you’re officially not a Geek then?
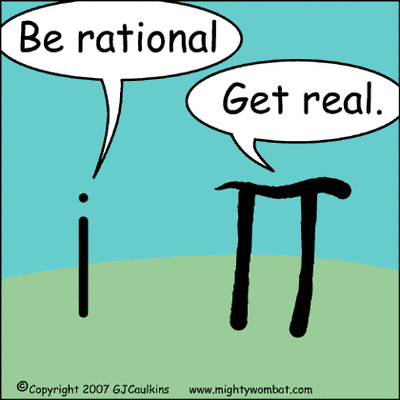
i, in Maths and Physics stands for imaginary numbers.
Unless you read Maths or Physics at University, (or sat the Irish Leaving Certificate Higher Maths), if somebody mentioned imaginary numbers, you probably would think they were making it up. If you did study ‘Complex Numbers’ you would know that they were!
In the once upon a time of ‘pre Complex Number Enlightenment’ we were told that you can not find the square root of a negative number. [At this point, if you are not sure what a square root is, quit while you are ahead and come look at some ultra-cute platypusses instead!]
Around the time of the Reformation, (October 31st 1517 apparently) some Italian Mathematicians realised it had been stupid to say you can’t find the square root of a negative number, because if you multiply the square root of a negative number (e.g. √-5) by itself (√-5) then you have a negative number (in this e.g. -5) …. so what was the problem!
Not wanting to upset all those mathematicians who before 1517 had said that square roots of negative numbers could not be found, the mathematicians decided to call the square root of negative numbers ‘imaginary numbers’ and gave them the letter i such that √-1 = i (You now also have the first part of the cartoon joke above)
In some circles that physicists and mathematicians customarily circumnavigate, i has already got a use, and hence those reprobates who used i for current (better known as Engineers) had to use a silly letter for imaginary numbers too. (they really had the point of using sensible letters all skewways) Engineers use the letter j. They would therefore also be expected to call an iPhone, a jPhone, silly people that they are!
As for the cartoon at the top of this post, well ∏ (the Greek letter pi) is the special number that enables us to calculate the circumference or area of a circle knowing only the radius. [Feeling dizzy….. click here] The exact value for pi is still not known…3.14159265359….. but it has been calculated to 10 trillion decimal places (10,000,000,000,000). Because the exact value is not known, the number goes on forever and is therefore known as an irrational number. Ta-Da!
If you got all that… you might be interested in the song….
An imaginary number (eg 5i) can be added to a real number (eg 7) to form a complex number of the form 7 + 5i , where 7 and 5 are called, respectively, the real part and the imaginary part of the complex number
A song about complex numbers is introduced annually to first year physics students at Royal Holloway University of London, by the Sonorous Dr Stuart Flockton to the tune of John Brown’s Body
Mine eyes have seen the glory of the Argand diagram
They have seen the i’s and thetas of De Moivre’s mighty plan
Now I can find the complex roots with consummate elan
With the root of minus oneComplex numbers are so easy
Complex numbers are so easy
Complex numbers are so easy
With the root of minus oneIn Cartesian co-ordinates the complex plane is fine
But the grandeur of the polar form this beauty doth outshine
You be raising i+40 to the power of 99
With the root of minus oneYou’ll realise your understanding was just second rate
When you see the power and magic of the complex conjugate
Drawing vectors corresponding to the roots of minus eight
With the root of minus one
Post Script: My friend Ian, who is a fully qualified geek, has qualified my definition or rational and irrational numbers on facebook. Here’s his comment;
A definition for rational numbers, for example 1/7, 4/5 or 3/61 is that the decimal sequence will repeat after a given number of digits. 1/7 =0.142857142857… . Note 6 digits. A little geeky fact is 2/7= 285714285714… notice the sequence is the same it just starts at 2 this time instead of 1. 3/7= 0.428571, etc etc. 1/13, 2/13, 3/13,… is an interesting one; so is 1/17, 2/17, 3/17,…. Irrational numbers do not have a repeating sequence. Pi is the one best known so after 10,000,000,000,000 decimal places there is no repeating sequence. There is never a repeating sequence it goes on ad infinitum it is just that computers do the hard work and this is as far as they have got so far.










Pingback: You are a geek if you get this…… « Day One
Do I have to do an exam now?
LikeLike
I got Peter to read this to check if it read sensibly to a non-physicist, and he told me that a joke was no good if you had to explain it. So that makes this post pretty redundant and therefore you have been let off the exam thanks to Peter :).
I was going to upload a video of our class singing the Complex Number song, but that wouldn’t have added anything I don’t think, and probably was more painful than funny unless you were in the video. I couldn’t find the video anyway, so the blog world has been saved for another day!
LikeLike
Phew what a relief! maths and I don’t go beyond fingers and toes!
LikeLike
🙂
LikeLike
Huh? I mean , really, huh?
LikeLike
Lol, I went looking for this post as an explanation for the ‘geek’ one for you. Oh well. I’ll explain it when I see you!
LikeLike
As I said to Auntie Marie (Grannymar) ;
I got Peter to read this to check if it read sensibly to a non-physicist, and he told me that a joke was no good if you had to explain it. So that makes this post pretty redundant and therefore you have been let off the exam thanks to Peter .
LikeLike
Damn. I wanted to take it. I’ve been studying hard and I think I’ve figured out how to travel faster than the speed of light so time travel will be possible. Oh well, I’ll just keep it to myself.
LikeLike
I could send you the test paper, but time travel isn’t on the test, sorry 😦
LikeLike
Reblogged this on verbotenedunkelheit.
LikeLike